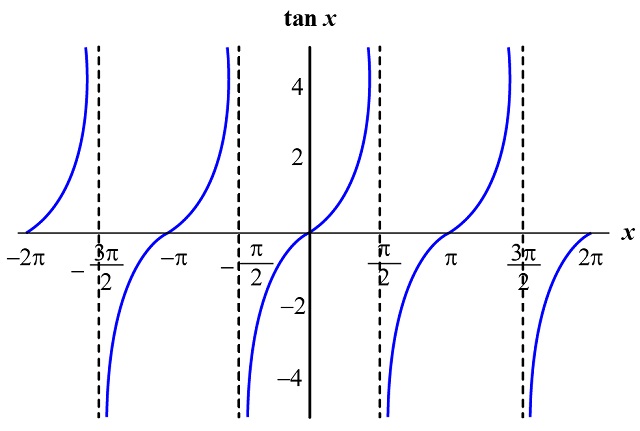
INTRODUCTION
Tan 0 degrees is a trigonometric function. That tells us the ratio of the length of the opposite side of an adjacent triangle. And while we are talking about it. Then we are talking about the angle of 0 degrees which is made with the x-axis. Let’s see it in simple words, It is equal to 0. It means that we are talking about the right-angle triangle. In which one of the angles is 0 degrees. And also the ratio of the angle of the adjacent side is 0. In simple words, it is a triangle essentially a straight line along the x-axis. So, in summary, We can express it in degrees is 0, indicating a horizontal line along the positive x-axis. So let’s look at it properly in this blog.
Tan 0 degree
The value of it is Zero. Also, we can write it in the radian as tan (0° × π/180°), tan (0π), or tan (0). In this article, we are going to learn about it more thoroughly and also with the help of an example. Here are some examples:
- Tan 0°: 0
- Tan (-0 degrees): 0
- Tan 0° in radians: tan (0π) or tan (0 . . .).
What is the Value of Tan 0 Degrees?
The value of it is 0. We can also define the tan 0 degrees as the angle equivalent to (0 degrees ) and in the radians (0…0)
Hence we know that if we have to convert degrees into radians we have to use
Radians == θ in degrees × (pi/180°) and also if we have to degree into the radians then we have to use 0 degrees = 0° × (π/180°) rad = 0π or 0
Hence tan 0° = tan(0) = 0
Explanation:
We are talking about the angle that lies in the x-axis in a positive direction. Hence the value of the tan 0° value = 0
Since the tangent function is periodic, we can represent tan 0° as, tan 0 degrees = tan(0° + n × 180°), n ∈ Z.
⇒ tan 0° = tan 180° = tan 360°, and so on.
Note: Tangent is an odd function, Then we can say that the value of the tan(-0°) = -tan(0°) = 0.
The method was used to find the value
We can find the value using the :
- By the use of the trigonometric function.
- And also by using the unit circle.
Let’s see Tan 0 in the Trigonometric function
By the use of the trigonometric formula, We can also express it. Hence the list of the formulas is mentioned below:
- sin(0°)/cos(0°)
- ± sin 0°/√(1 – sin²(0°))
- ± √(1 – cos²(0°))/cos 0°
- ± 1/√(cosec²(0°) – 1)
- ± √(sec²(0°) – 1)
- 1/cot 0°
Important: Hence it lies in the positive X-axis. Then we are getting the final value of it in degree is 0.
We can also use the trigonometric identity to express it in degrees such as:
- cot(90° – 0°) = cot 90°
- -cot(90° + 0°) = -cot 90°
- -tan (180° – 0°) = -tan 180°
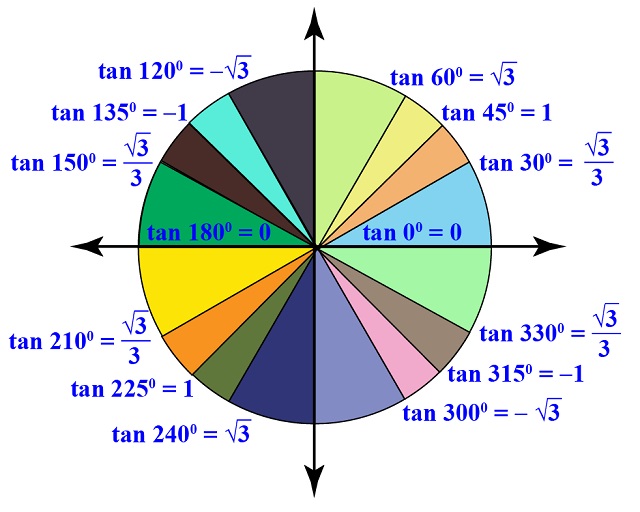
Expressing it by using the Unit circle
We can find the value of it by the use of a unit circle in the following way
First, we have to draw the radius of the unit circle ‘ r ‘ and form the 0-degree angle with the x-axis.
Hence it is in degrees equals the y – coordinates (0) and it is divided by the x – coordinates (1). And it forms the intersection point at (1,0) of a unit circle.
So the value of it in degrees = Y/x = 0
Conclusion
In conclusion, the tangent of the 0 degree. Is get denoted by tan(0°), and it is equal to 0. Hence it means that we are dealing with the right angle triangle. The one angle of the triangle is 0 degrees. And which forms the straight line along with the x-axis. However, in the radians, we can express it in degree as tan(0π). Hence the trigonometric function can be understood using various formulas. And that can be represented as in a circle. Also, it corresponds with the y- y-coordinate and is divided by the x-coordinate. And in the result, we get the value of 0. Hence simply it is a degree is zero. Hence it represents the horizontal line along with the x-axis.